Made in Humane Club
“For the past 3 years our (now) 12yr old son, Vidur, has been setting up a game stall at our society Diwali mela.
While his objective was to have fun, I had a selfish motive.
To teach him about money.
This year he earned 12K in profit.
And he can’t believe it!
1st year lesson: Sales
3 years back I wished for him to learn sales.
How to drive conversions?
What to write on the cardboard?
How to select the prizes?
How to price?
He ran a “break the can pyramid with 3 balls” game.
Priced it at Rs. 10 for 3 balls.
Made a sale of Rs. 4,000.
Had no idea the cost of the prizes he gave away, or something called profit.
But understood that he could have charged more.
Classic startup haha!
2nd year lesson: Costs
Last year, I wished for him to learn costs.
Fixed costs (stall cost, game cost etc) and variable costs (prizes).
He had a budget to work with.
He didn’t like that one bit.
“Why can’t we give away cool prizes?”
“Because they cost money, Vidur. And you only have so much to spend.”
He ran the same game.
Made a sale of Rs. 8,500.
Spent Rs. 5,000 in prizes and Rs. 3,000 in fixed costs.
He thought he made Rs. 8,500.
Cute! :))
3rd year lesson: Profits
This year, I wished for him to learn how to make money.
And I saw a different Vidur at work!
He decided on a new game.
Players had to throw table-tennis balls into 10 cups arranged as a cone, each with a score 1-10.
You total the score and basis that win prizes.
I asked him how much he wanted to make, in profit percentage?
He said atleast 75%
Wow!
I said, ok – let’s play excel excel :)))
We made an excel sheet.
Built the numbers.
I could see his mind work.
Sales:
His game pitch was “Earn Rs. 15,000”
How?
Score more than 30 points in 3 or 4 tries and pick any of the following prizes – which included a Marshall speaker for Rs. 15,000 (he didn’t buy it – we already had one).
He priced 3 tries for Rs. 20, 4 tries for Rs. 40.
Costs:
He wanted most people to walk away with a prize, however small.
So he set 5 slabs
1-5 points: Lollipop (worth Rs. 5)
6-10 points: Frooti (worth Rs. 10)
11-20 points: Frooti + Oreo (worth Rs. 20)
21-30 points: Coke + Oreo + Kitkat (worth Rs. 30)
30+ points: Pick from the grand prizes
He went about buying all of these, on a returnable basis from the local shop (very smart of him to consider returnable).
Invested 2,700 to do so.
Plus 2,000 for the stall.
Plus 500 for misc setup.
Profits:
He was on a roll that day.
I was the cashier handling the money.
He was the one selling and making people play.
Enticing them to go for 4 tries (for Rs. 40).
Celebrating each ball dropping into a cup.
Mid-way he realized the game was harder than he thought it would be.
So he encouraged the same people to play again, because it is a skill game eventually.
It was wonderful to see him in action.
End result:
Sales: Rs. 16,600 (40% went for 4 tries!)
Costs:
- Rs. 2,500 fixed
- Rs. 2,200 prizes
Profit: Rs. 11,900
What did he do?
Kept 1,900 with himself and asked me to invest 10K
How did he feel?
On top of the world.
He can’t believe he did it.
While he gets a fair bit of money on his birthday and other family events, this is the most he has made on his own.
And I don’t think anything else will come close to making this money feel sweeter!
PS 1:
He has been investing nearly all the money he has got till date, through me.
Has invested 1.05L so far, which has reached 1.23L at an XIRR of 24%
PS2:
This was the excel sheet we made :))
https://t.co/B7ipKKvMwi
Source: https://twitter.com/warikoo/status/1723989015723167848
Copied
Children are always bursting with questions. But, let’s be real, we didn’t always have the answers or the time to spare. So, we introduced Sabi to ChatGPT+. Here’s how it played out:
- Safety first. We kicked off chats with: “Hi, I’m Sabi. I’m a seven-year-old in second grade. Can we talk?” This let ChatGPT know a young mind was on the other end.
- Quenching curiosity. Sabi dived into everything from “How did the universe begin?” to “How do you play with 1-year-old babies?” Sure, some replies were cliche, but to a seven-year-old, it’s fresh info.
- Intellectually active. Instead of just zoning out in front of the TV, she posed questions, read back, and thought of what to ask next.
- Better screen time. Suddenly, those hours in front of a screen weren’t just passive; they were educational.
- Typing skills. Introducing her to a laptop for this meant she got a head start on keyboard skills.
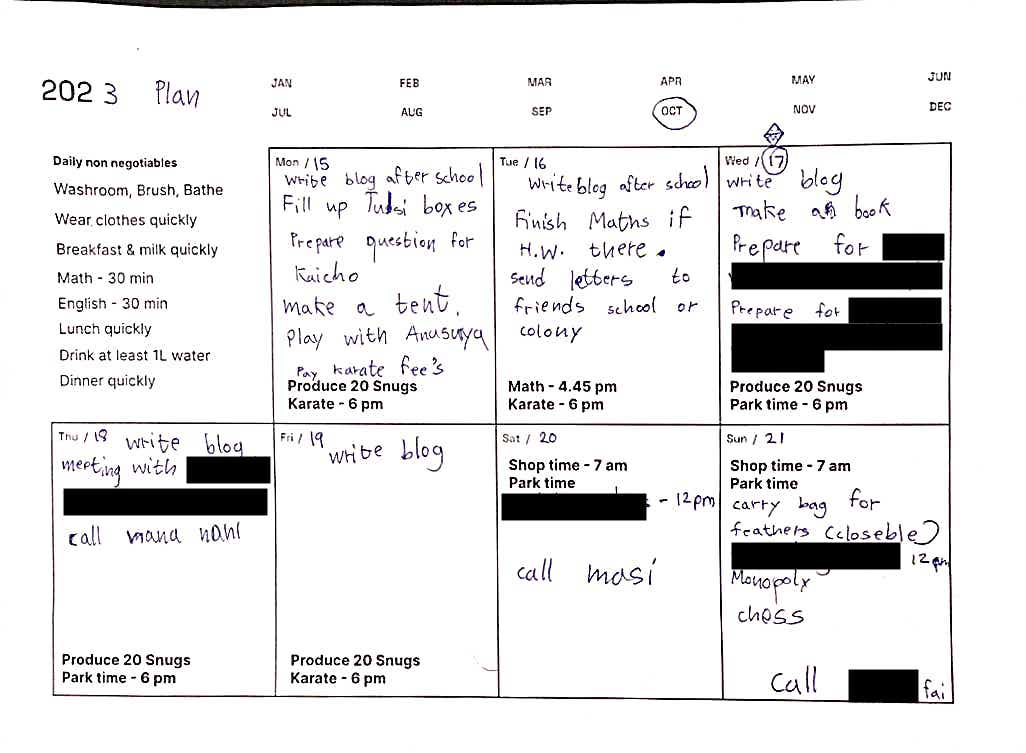
CopiedWhen raising Sabi, we often faced a common question: “How does Sabi juggle so many tasks at 7?” Our method? Think about how you organize your days as young adults, and we did something similar for her.
We have mass-printed weekly planners for Sabi. On the left side, we outlined essential tasks—like brushing teeth or practicing math. Beyond that? She got to fill in the rest, mapping out her week.
Each week, she used two sheets. Every Sunday night, she sketched out her ideal week. Then, as each day passed, she noted what she really did on a second sheet. She’d review her notes every morning, setting the tone for her day.
Notice the phrase “prepare for”? The planner has inculcated the habit to not just show up, but to plan ahead and make the most of from showing up.
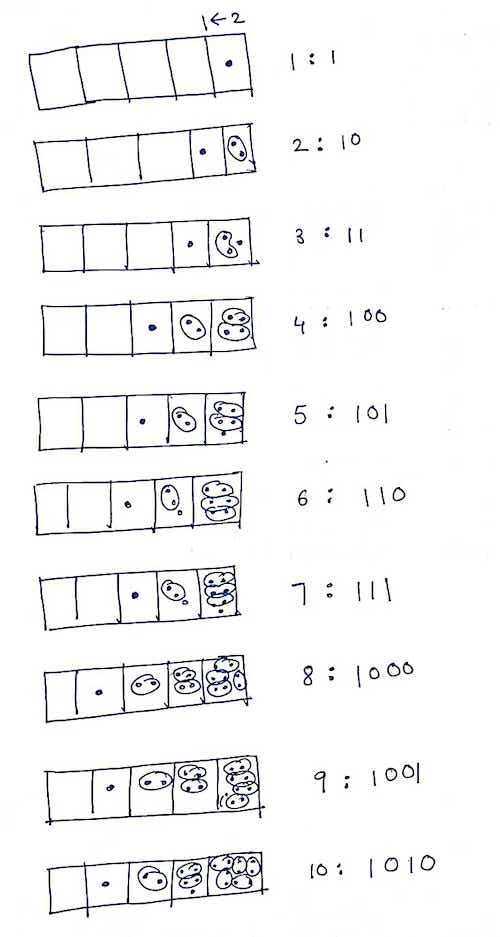
1CopiedImagine a machine which is nothing more than a row of boxes that extends as far to the left. Let’s call it a “two-one machine” both written and read in a funny backwards way.
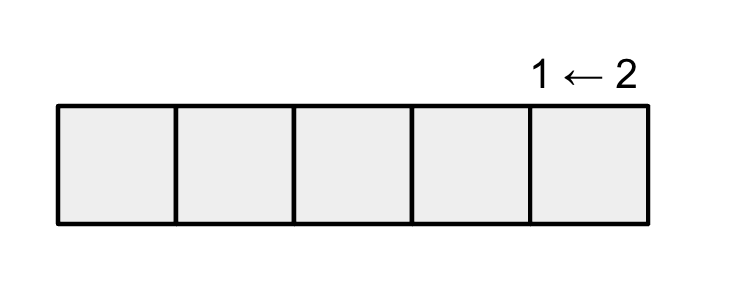
And what do you do with this machine? You put in dots. Dots always go into the rightmost box.
- Put in one dot, and, well, nothing happens: it stays there as one dot.
- But put in a second dot – always in the rightmost box – and then something exciting happens. Whenever there are two dots in a box they explode and disappear – Bhoom! – to be replaced by one dot, one box to the left.
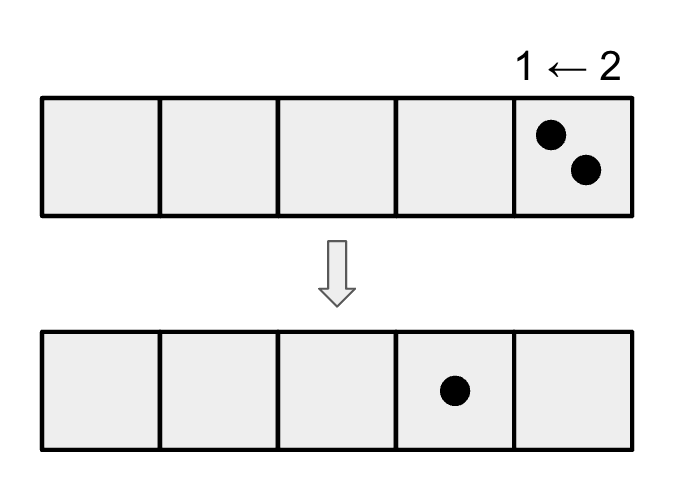
We see that two dots placed into the machine yields one dot followed by zero dots.
Putting in a third dot – always the rightmost box – gives the picture one dot followed by one dot.
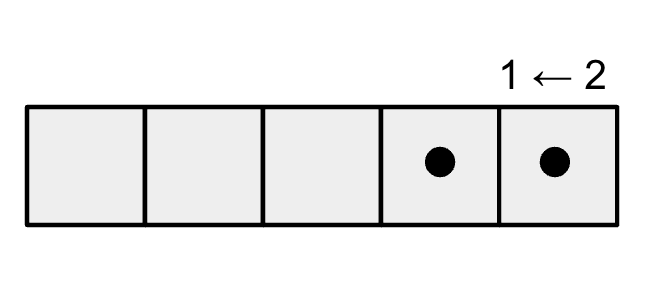
Let’s make a table and do it for all numbers till 10
| Numbers | 1 <- 2 |
|---|---|
| 1 | 1 |
| 2 | 10 |
| 3 | 11 |
| 4 | 100 |
| 5 | 101 |
| 6 | 110 |
| 7 | 111 |
| 8 | 1000 |
| 9 | 1001 |
| 10 | 1010 |
Here is a visual way to look at it
Now, instead of playing with a 1 <- 2 machine, we could play with a 1 <- 3 machine (again
written and read backwards, a “three-one “machine). Now whenever there are three dots in a
box, they explode away to be replaced with one dot, one box to the left.
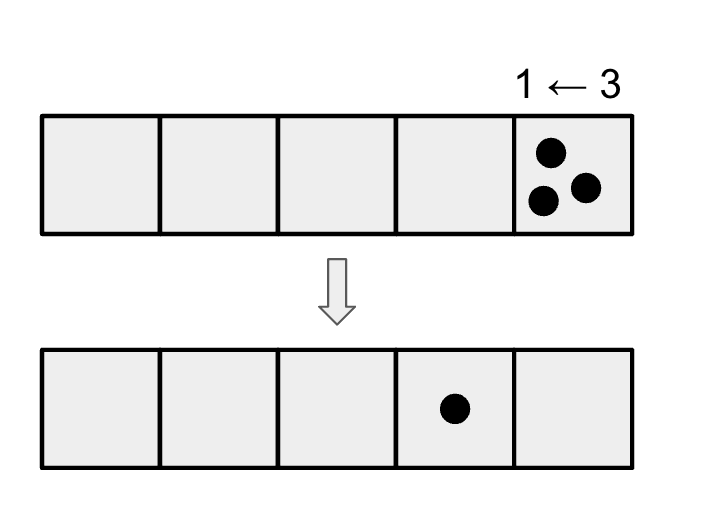
| Numbers | 1 <- 3 |
|---|---|
| 1 | 1 |
| 2 | 2 |
| 3 | 10 |
| 4 | 11 |
| 5 | 12 |
| 6 | 20 |
| 7 | 21 |
| 8 | 22 |
| 9 | 100 |
| 10 | 101 |
You can try this for different types of machine: 1 <- 4, 1 <- 5 and so on…
What are these machines doing?
Can you figure out what these machines are actually doing? Why is the code for two hundred and seventy-three in a 1 <- 10 machine, “273”? Are all the codes for numbers in a 1 <- 10 sure to be identical to how we normally write numbers. If you can answer that question, can you then also make sense of all the codes for a 1 <- 2 machine? What does the code 1101 for the number thirteen mean?
Both the above approaches, teaches the concept of binary (1 <-2) , tertiary (1 <- 3) and decimal (1 <- 10) number systems.
CopiedPuzzle: Consider you have squares of different sizes 1cm, 2cm, 3cm, 4cm and 5cm. How many minimum number of squares can be used to cover 7 x 7 matrix?
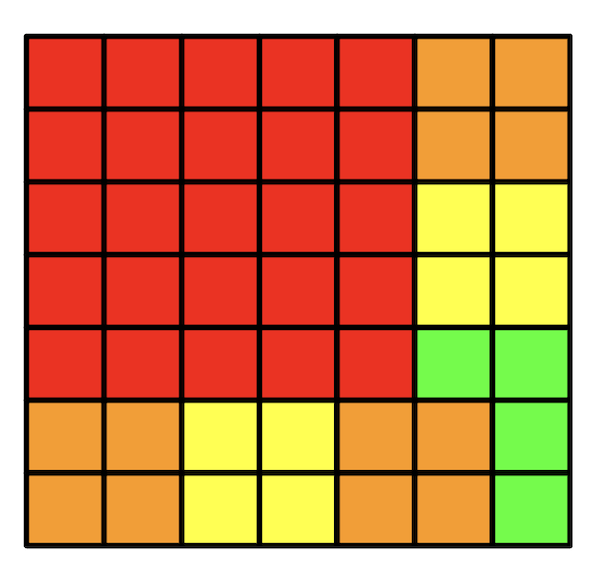
The most common answer that you will end up easily by trying different combinations of squares is 10. However, there are less than 10 squares that can fit it. Here’s the solution for it:
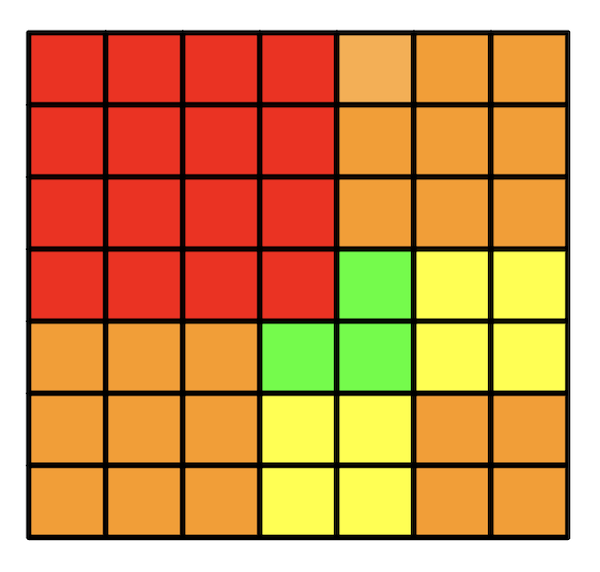
CopiedThere are patterns even in daily objects arounds us. A book’s or any grocery product barcode may look like a bunch of random digits, but there is a secret mathematical code hidden in the barcode.
What pattern do you see in the Barcode below?
- There are alternate black and white vertical stripes
- Some stripes are thick and some stripes are thin
- It starts and ends with black color stripe.
Puzzle: Consider a 10cm barcode. How many different patterns can you create using combinations 1 cm or 2 cm (black and white stripe)
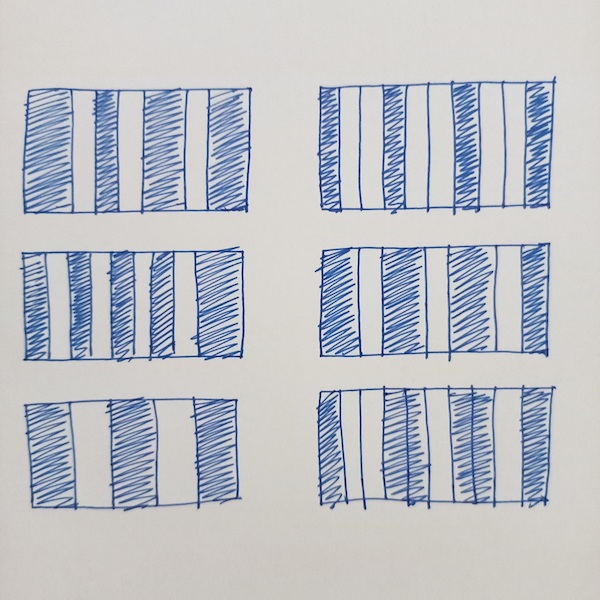
You can use paper cut outs of 1 cm and 2 cm and then place them in different combinations to find the patterns.
CopiedWhen Sabi was younger, we encouraged her independence. Simple tasks like choosing outfits or tying shoe laces were hers to tackle. One day, Sabi attempted to comb her hair, which is soft and tends to frizz. A mishap led to a tangled rubber band and we had to snip a bit of her hair. We advised her to wait until she turned 10 to tie her hair again. Yet, her curiosity persisted.
Last October, when her aunt inquired about a gift, Sabi’s initial thought was dolls. This took us aback, since dolls never truly caught her interest. A moment later, she wondered aloud if there were dolls with lifelike hair and accompanying combs. Sabi wanted to comb the doll’s hair to learn how to comb her own hair.
Copied
What is the difference between the two leaves?
- Gulmohar has Rounded leaves while Jacaranda has Pointed leaves
- …
- Gulmohar leaves are in pair of two, while Jacaranda leaves are in pairs of two with one single leave at the tip of the stem. Hence, Gulmohar leaves are 2n and Jacaranda leaves are 2n+1.
Copied